Molecular Energetics Group

Thermogravimetry or thermal gravimetric analysis (TGA) is used to measure weight changes in a sample, in a controlled atmosphere, as a function of temperature or time. These changes can be associated to process such as chemical reactions, solvent and water evolution, and Curie point transitions.

The apparatus available at the Molecular Energetics Group is TGA 7 from Perkin-Elmer (Figure 1). It mainly consists of a balance with a resolution of 1 mg, and an electrically heated tubular oven, that surrounds a platinum pan containing the sample. The pan is suspended from one arm of the balance and its bottom is positioned close to a thermocouple that measures the temperature. The balance chamber is normally kept under a positive flow of nitrogen and the furnace is purged with helium to continuously remove the gases evolved from the sample. A computer is used to control the instrument.

Figure 1. TGA 7 apparatus from Perkin-Elmer.

 

Analysis may be carried out isothermally or by raising the temperature of the sample at a constant rate. The primary experimental result is a plot of the weight loss against time or temperature.

The mass scale of the instrument is normally calibrated with a standard 100 mg weight and the temperature calibration is based on the measurement of the Curie points (T_C) of alumel alloy (T_C = 427.35K) and nickel (T_C = 628.45 K) standard reference materials.

The apparatus has been mainly used to obtain the temperature onset for decomposition and the stoichiometry of hydrates and solvates, and to study the kinetics of the dehydration/desolvation processes.

The general procedure for obtaining the stoichiometry of and hydrate or solvate can be exemplified by the study of the 4-hydroxynicotinc acid hemihydrate, 4HNA•0.5H2O [1]. The dehydration of a sample with an initial mass mi = 1.650 mg at a heating rate Β= 5 K/min led to the curve in Figure 2.

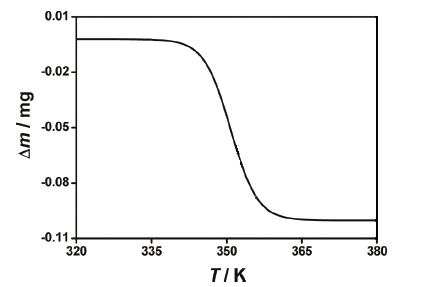
Figure 1. TG measuring curve.

From this curve it is possible to conclude that the temperature onset of dehydration is 344.8 K and the mass loss Δm = 0.103 mg. The amount of substance n of H2O corresponding to 1 mol of 4HNA is given by:

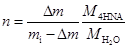(1)

where M_4HNA = 139.1088 g/mol and M_H2O = 18.0153 g/mol are the molar masses of anhydrous 4-hydroxynicotinc acid and water. The obtained results lead to

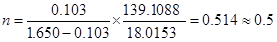(2)

The mean value of the results of five such experiments led to n = 0.51±0.02 in good agreement with the hemihydrate stoichiometry that was also corroborated by single crystal X-ray diffraction analysis.

Thermogravimetric studies also allow the determination of kinetic data [2]. Indeed the extent of conversion, a, at a given time, t, may be obtained as:

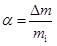(3)

where mi is the initial mass and Δm is the mass loss corresponding to t. The rate constant, k, for the process may be obtained from a rate equation:

(4)

where f(a), dubbed the reaction model, is a function describing the mechanism of the process. The value of k and the explicit form of f(a) can both be obtained through a fit to the experimental a vs. t data.

By performing isothermal experiments at different temperatures it is possible to obtain the temperature dependence of the rate constant. This is turn affords the Arrhenius activation energy, Ea, and its companion pre-exponential factor, A, by fitting the equation:

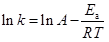(5)

to the experimental data, or the corresponding molar enthalpy, Δ^‡H_m, and entropy, Δ^‡S_m, of activation based on and Eyring plot:

(6)

In equations 5 and 6, T is the absolute temperature, R is the gas constant, kB represents the Boltzmann constant, and h is the Planck constant.

It is also possible to perform non-isothermal kinetic experiments by thermogravimetry but these are normally regarded as less reliable than the isothermal studies.

Go to top